Web site under construction. Most links don’t work.
Ken Brakke
Professor Emeritus
Department of Mathematics
and Computer Science
Susquehanna University
Phone: 570-495-0452 (mobile)
Email:
brakke@susqu.edu
Snail mail: 2603 Pacific Highlands Ave, Ferndale, WA 98248
|
|
The Surface Evolver
Version 2.70, August 25, 2013
My Surface Evolver is an interactive program for the modelling of liquid surfaces shaped by various forces and constraints. The program is available free of charge.
Book on Microdroplets, using Surface Evolver
Jean Berthier and I have collaborated on a book about the behavior of liquids in microfluidic circumstances. Over a hundred Evolver models, available for download from the book’s website (if you buy the book).
Book on Open Microfluidics, using Surface Evolver
Jean Berthier and I have done another book, about liquids flowing in channels that are not completely enclosed. Includes lots Evolver models, available for download from the book’s website (if you buy the book).
Plateau’s book translated into English
The result of my amateur attempt to translate Joseph Plateau’s famous 1873 book on soap films and surface tension.
Random Fractals
A gallery of random fractal images generated with iterated function systems (IFS), along with my own applet to generate more. Infinite complexity and amazing variety from simple rules!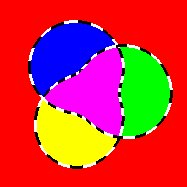
Polycut
A program for visualizing multiple universes connected by gateways formed by cosmic strings. The image shows five universes (with different color skies) connected by a string in the shape of a trefoil knot. Polycut reveals how soap films are least-area boundaries between universes.
Beating Kelvin’s partition of space.
What is the least area way to partition space into unit volumes?
The wet X.
A Java applet showing the equilibrium states of a 1-dimensional soap film spanning the corners of a rectangle with liquid in the interior of the film.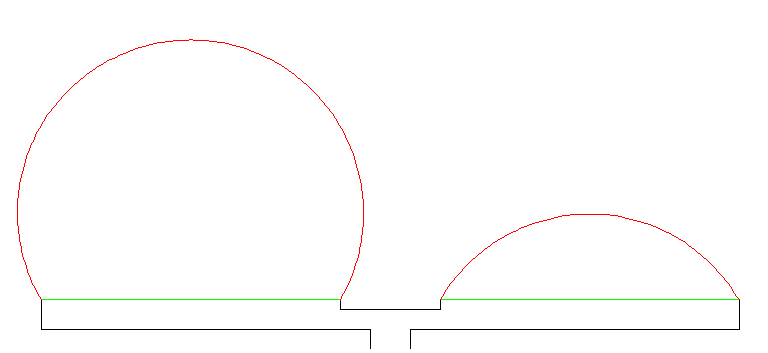
The Double Bubble Pipe.
A Java applet showing the stable equilibrium states of a multiple-bubble pipe. Illustrates how joining stable systems can result in an unstable system.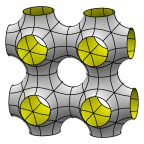
Triply periodic minimal surfaces
Surfaces of zero mean curvature that repeat periodically in three dimensions.
Soap film cones
Which soap films on wire frames form perfect cones straight to the center?
There are surprisingly few.
Soap films on knots
Knotted wires make for some very interesting soapfilms!
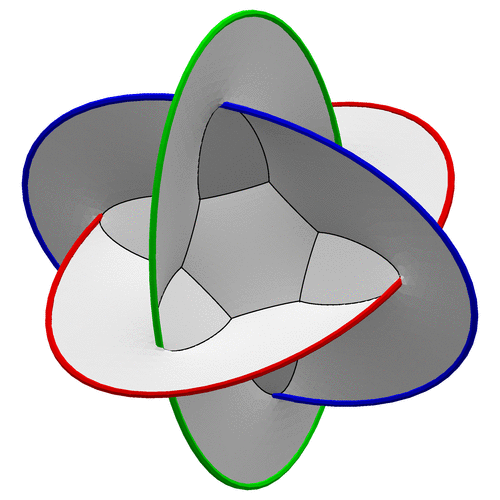
Soap films on the Borromean Rings
The Borromean Rings are three rings linked so that any pair of rings are
not linked, but all three are (i.e. cannot be pulled apart). The Rings
support many soap films.
Go here for static images.
For 3D mouse-spinnable images, go
here.
And for better 3D mouse-spinnable images using the upcoming WebGL 3D
technology, go
here. (Only works on FireFox 4 Beta or later,
suitably configured. Before loading, browse “about:config” and
set “webgl.enabled_for_all_sites” to “true”; this setting will be
permanently remembered.)
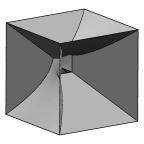
The Opaque Cube Problem
What is the least area surface that can block any ray of light from
passing through the interior of a cube?
Statistics of Space Aliens
Even if the galaxy potentially has millions of space-faring civilizations,
the first such civilization probably gets about a
100 million year head start on the second. We look to be the first,
so the galaxy is ours to colonize without opposition!
My papers.
Grain growth movie.
Ruler and compass constructions.